Earth Geometry
Earth geometry is a mathematical concept that deals with the spherical Earth in relation to distance, time and location of places which are found on the latitude or longitudes
« Previous Next »Earth Geometry Fundamentals
The Earth is made up of two demarcation of line namely the latitude and the longitude
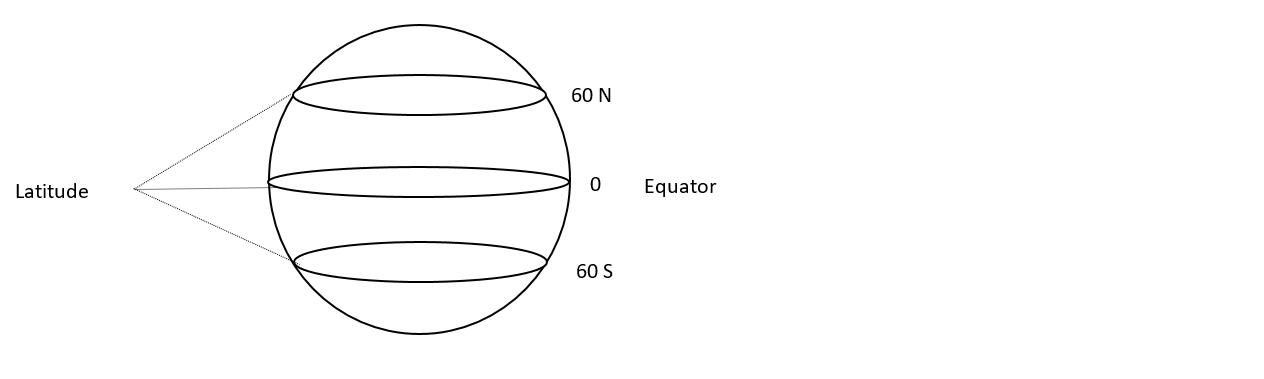
what you should know about the latitude
latitude: are lines which run from east to west of the earth
The latitude which is 0 degrees is refered to has the equator
The signs for the line on the latitudes are N (north) or S (south)
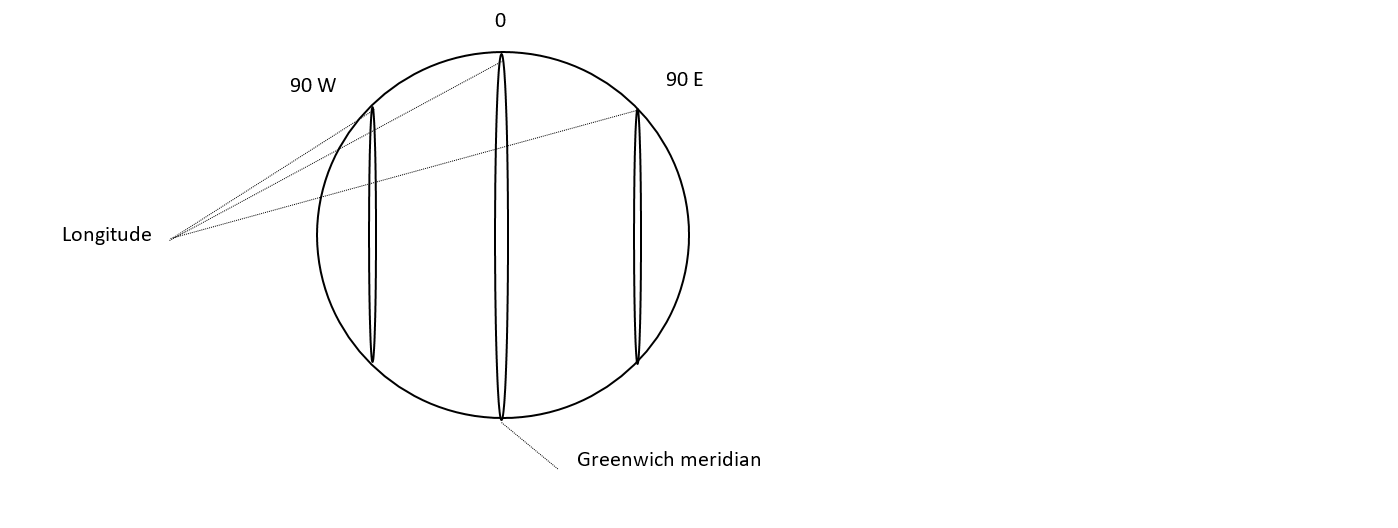
what you should know about the longitude
longitude: are lines which run from north to south of the earth
The longitude which is 0 degrees is refered to has the Greenwich meridian
The signs for the line on the longitude are E (east) or W (west)
How to Calculate Time in earth Geometry
1. First step to take to calculate the time between two difference places is to find the difference between those two places which can be the longitudes or latitudes
2. when finding the difference between two places you have to know when to add or subtract two points:
if the locations are found in the same region you have to subtract those two places example of places which are: E and E and given that the time at E is 12:00 hrs what is the time at E
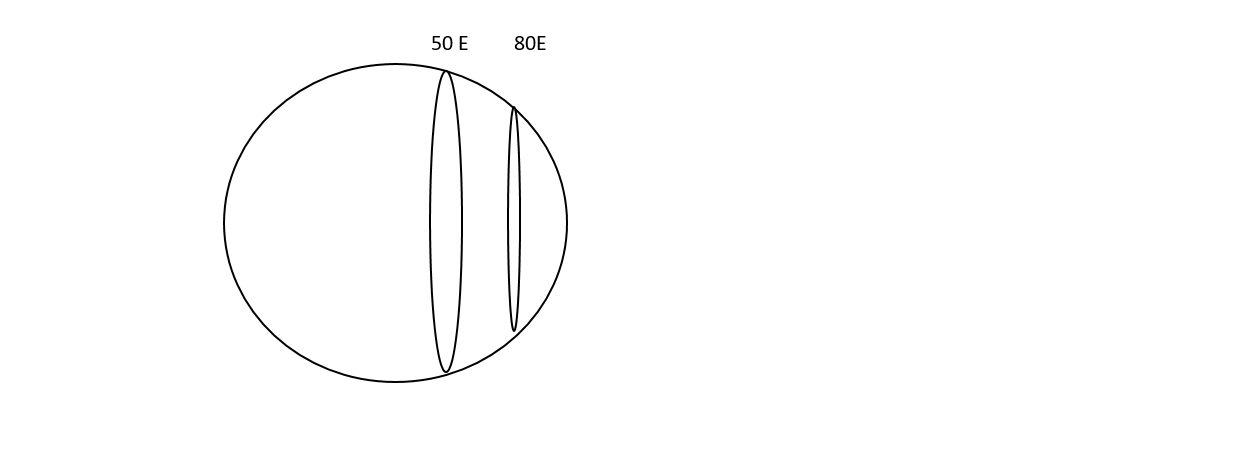
The difference between the two places:
difference = -
difference =
hours =
hours =
Therefore time at E is 12:00 + 2:00 = 14:00 hrs
if the places are in difference locations the difference is found by adding the values of those two places example are: W and E

Given that the time at E is 18:00 hrs what is the time at W
difference = +
difference =
hours = = 9.33
= and a remainder of
= 4 minutes
= x minutes
x = 9 minutes
09:09 hrs
The time of the place which is in the west having W is 18:00 - 09:09 hrs = 08:51 hrs
Points to note
1. To find the time of a place which is in the east, given that you have been provided with the time of a place in the west. The time difference is added to the time of a place in the west to find time of a place in east
2. Secondly to find the time of a place which is in the west, provided that you have been given the time of a place in the east. The time difference is subtracted to the time of a place in the east to find time of a place in west
3. is equal to 1 hour
4. Fourthly is to divide into the difference find between the latitude or the longitudes
5. if they are any remainders when dividing with take note that the remainders are minutes.
6. is equal to 4 minutes therefore multiply the remainder with 4 to find the minutes
Example 1
In the diagram, find:
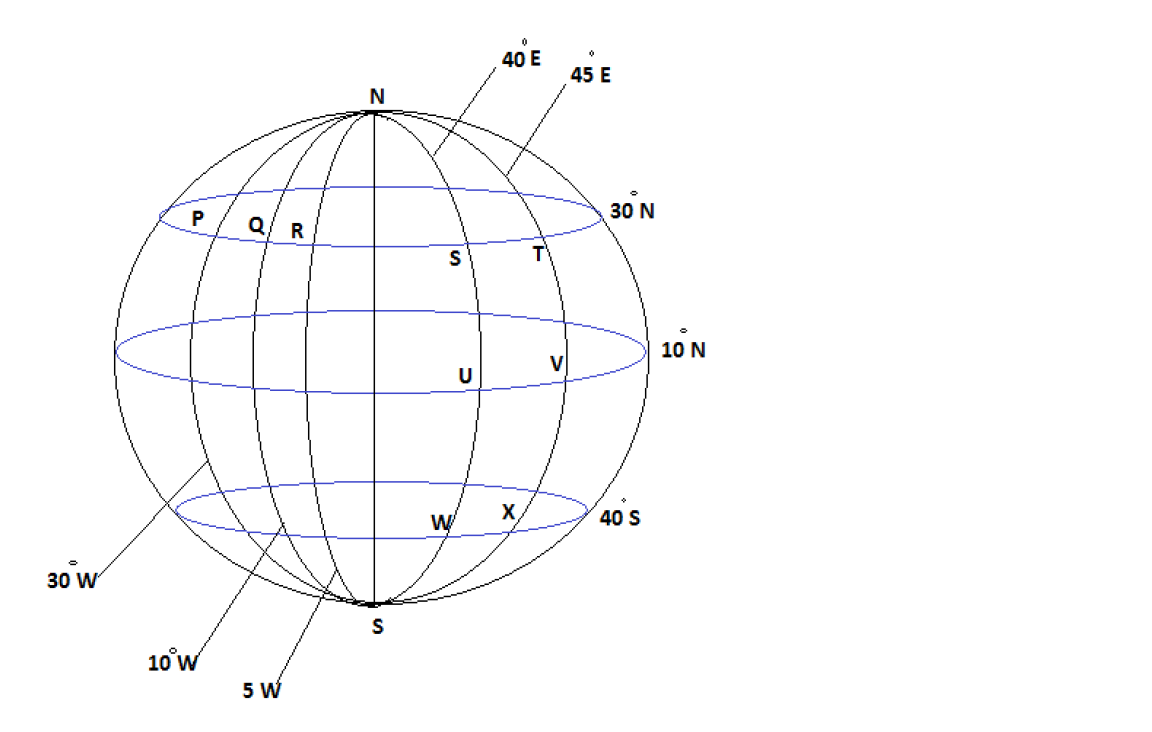
(i) Describe position Q, W and V
(ii) The difference in longitude between P and Q ; P and T
(iii) The difference in latitude between S and U; S and W
Solutions
(i)
Q = N, W
W = S, E
V = S, E
(ii)
P and Q
P = W, Q = W
P and Q = W - W
P and Q =
p and T
p = W, T = E
p and T = W, + E
p and T =
(iii)
S and U
S = N, U = N
S and U = N - N
S and U =
S and W
S = N, W = S
S and W = N + S
S and W =
Example 2
W, X, Y and Z are four points on the surface of the earth as shown in the diagram below.
[𝜋 = 3.142,𝑅 = 3437𝑛𝑚]
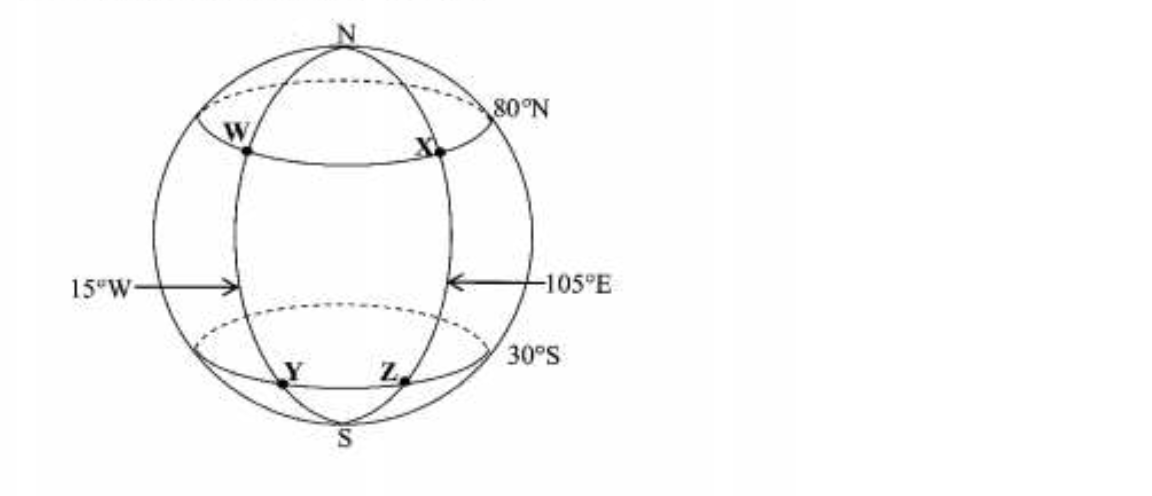
(i) Calculate the difference in latitude betweeen W and Y.
(ii) Calculate the distance in nautical miles between
(a) X and Z along the longitude 105⁰E
(b) Y and Z along the circle of latitude 30⁰S
Solution:
(i)
W = N, S
W = N, + S
Answer:
(ii)
How to find distance in earth geometry
(a)
Distance XZ
Formula
= 3.142, R = 3437nm, = 80⁰N + 30⁰S = 110,
Answer:
(b)
Distance YZ
Formula
Note that
R = r meaning that capital R (Radius) is equal to small r times cos(x)⁰. This is applied when finding distance betweeen latitudes
= 3.142, r = 3437nm, = 15⁰W + 105⁰E = 120, =
Answer:
Points to note
= theta represents the difference in latitude or longitude
R = represents the Radius
= Pi represents or 3.142
= Formula to find the distance between longitudes
= Formula to find the distance between latitudes
R = r
How to solve earth geometry
The diagram below shows three points P, Q and R on the surface of the earth.
[Take as 3.142 and R = 6370km ]
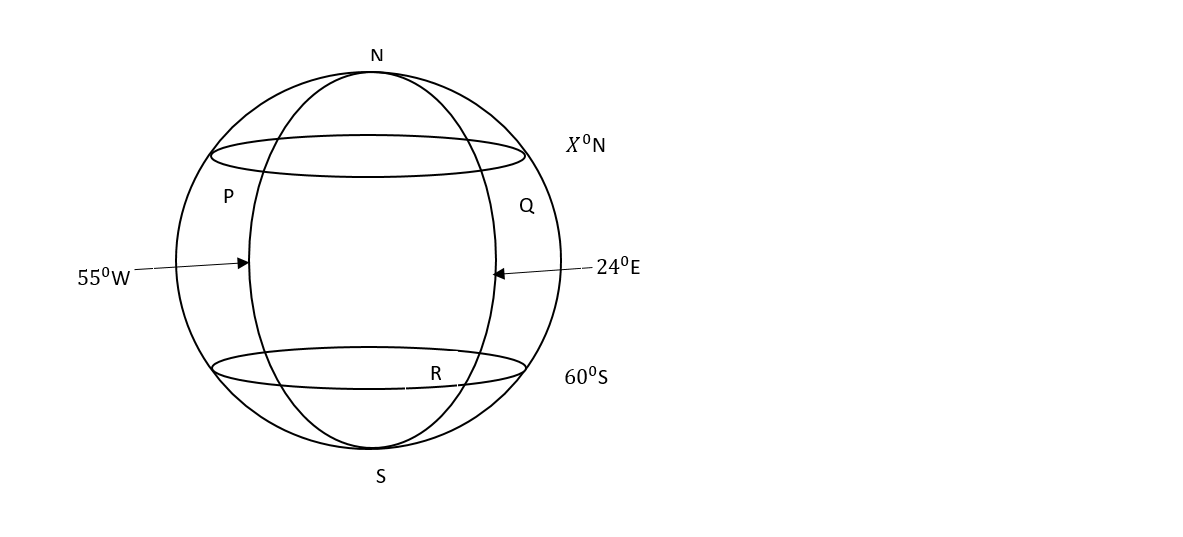
(a) Calculate the difference in longitude between P and R
(b) Given that the distance between P and Q on latitude is 4392.079611km, calculate X.
(c) Calculate the distance QR on longitude .
Solution:
(a)
P = 55 W
R = 24 E
difference =Answer:
(b)
Formula
D = 4392.079611
= 79
= 3.142
R = 6370
= ?
Answer:
(c)
Formula
QR = ?
Q = 60
R = 60
= 120
= 3.142
Radius = 6370
QR = 13,343.027
Answer: QR = 13,343.027 km