Chemical formulae and Equations

A chemical formula consist of a symbol or symbols showing the number of atoms in one molecule of an element or a compound

Steps to consider when writing the chemical formula

1. Write the symbol for the combining elements and radicals
2. Write the Valency of each element or radical at the top at its top right hand side
3. Exchange the Valencies of the combining elements and radicals by writing them at the bottom right hand side of the element or radical. If the number is 1, do not write it.

In some formulae, radicals are written in brackets followed by a small sub script digit.

Example 1

Write the formula for each of the following compounds

(a) Sodium chloride

(b) Potassium carbonate

Solution

(a) Na     Cl

= ${\mathrm{Na}}^1$     ${\mathrm{Cl}}^1$

Answer: NaCl

(b) K     ${\mathrm{CO}}_3$

= $K^1$     ${{\mathrm{CO}}_3}^2$

Answer: $K_2{\mathrm{CO}}_3$

State symbols

State symbols are letters that are used to show the physical state of substances in the equation State symbols are placed in brackets after the name or formula of each substance in the equation

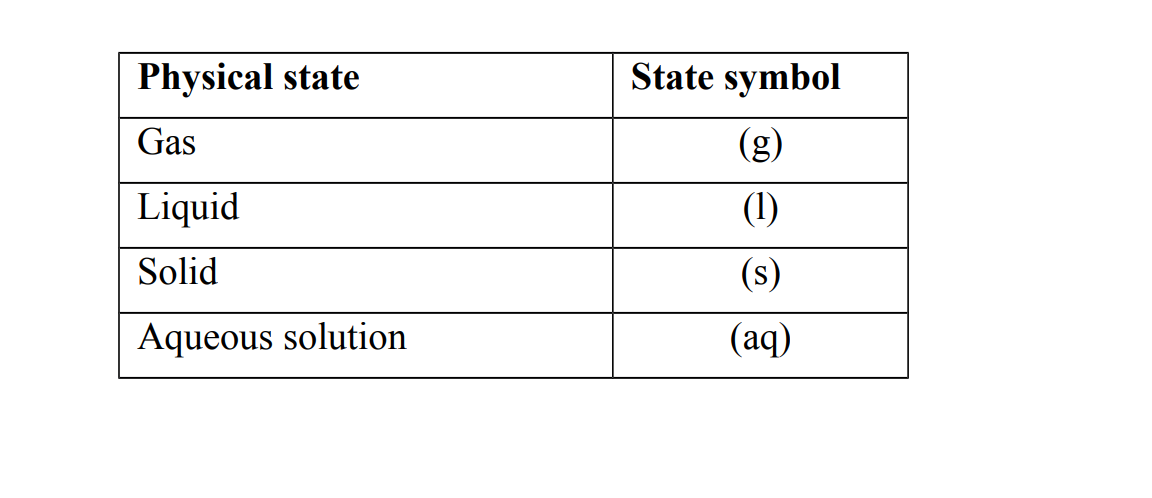
Equations

An equation is a chemical sentence which describes what is happening in a chemical reaction

An equation can be represented in the form:

A + B → C + D

The plus (+) sign on the left hand side means “react with”

The plus (+) sign on the right hand side means “and”

The arrow (→) between the reactants and products means “to form”

Word equations

Word equations are chemical equations written in words

Example 2

Write down the word equation for each of the following reactions including state symbols

(a) Magnesium metal reacts with oxygen gas to form magnesium oxide

(b) Hydrogen sulphide gas reacts with oxygen gas to form sulphur and water

(c) Iron (II) chloride solution and hydrogen gas are produced when iron reacts with dilute hydrochloric acid

(d) Iron reacts with chlorine gas to form iron (II) chloride

Solution

(a) Magnesium(s) + Oxygen(g) → Magnesium oxide(s)

(b) Hydrogen sulphide(s) + Oxygen(g) → Sulphur(s) + Water(l)

(c) Iron(s) + Hydrochloric acid(aq) → Iron (II) chloride(aq) + Hydrogen(g)

(d) Iron(s) + Chlorine(g) → Iron (II) chloride(s)

Equations with symbols

Writing balanced chemical equations

Balancing the equation is the process of making the number of each type atom equal on both sides of the equation

Example 3

Write down balanced chemical equations including state symbols for each of the following word equations

(a) Mercury oxide(s) → Mercury(l) + Oxygen(g)

(b) Hydrogen(g) + Oxygen(g) → Water(l)

(c) Magnesium(s) + Oxygen(g) → Magnesium oxide(s)

(d) Sodium(s) + Water(l) → Sodium hydroxide(aq) + Hydrogen(g)

(e) Calcium oxide(s) + Hydrochloric acid(aq) → Calcium chloride(aq) + Water(l)

Solution

(a) 2HgO(s) → 2Hg(l) + $O_2$(g)

(b) ${\mathrm{2H}}_2$(g) + $O_2$(g) → ${\mathrm{2H}}_{2}O$(l)

(c) $\mathrm{2Mg}$(s) + $O_2$(g) → $\mathrm{2MgO}$(s)

(d) $\mathrm{2Na}$(s) + ${\mathrm{2H}}_{2}O$(l) → $\mathrm{2NaOH}$(aq) + $H_2$(g)

(e) $\mathrm{CaO}$(s) + $\mathrm{2HCl}$(aq) → ${\mathrm{CaCl}}_2$(aq) + $H_{2}O$ (l)

Ionic equations

Ionic equations show only the ions involved in a chemical reaction

Ions not taking part in the reaction (spectator ions) are cancelled out in the construction of ionic equations

Steps to consider when writing the ionic equation

1. Construct a balanced chemical equation
2. Split only soluble ionic compounds into ions. Insoluble ionic compounds, elements and covalent compounds remain unchanged.
3. Cancel out spectator ions. These are ions that appear on both the left and right hand side of the equation.
4. Rewrite the equation without the spectator ions

Example 4

Write the ionic equations for the reactions below

(a) ${\mathrm{Ba(NO}}_{\mathrm{3)2}}$(aq) + ${\mathrm{Na}}_2$ ${\mathrm{SO}}_4$ (aq) → $\mathrm{Ba}{\mathrm{SO}}_4$ (s) + $\mathrm{2Na}{\mathrm{NO}}_3$ (aq)

(b) $\mathrm{NaOH}$(aq) + $\mathrm{HCl}$ (aq) → $\mathrm{NaCl}$(aq) + $H_{2}O$ (l)

Solution

(a) ${\mathrm{Ba(NO}}_{\mathrm{3)2}}$(aq) + ${\mathrm{Na}}_2$ ${\mathrm{SO}}_4$ (aq) → $\mathrm{Ba}{\mathrm{SO}}_4$ (s) + $\mathrm{2Na}{\mathrm{NO}}_3$ (aq)

= ${\mathrm{Ba}}^{\mathrm{2+}}$(aq) + ${{\mathrm{2NO}}_3}^{\mathrm{-}}$ (aq) + ${{\mathrm{2Na}}_{\mathrm{}}}^{\mathrm{+}}$ (aq) + ${{\mathrm{SO}}_4}^{\mathrm{2-}}$ (aq) → $\mathrm{Ba}{\mathrm{SO}}_4$ (s) + ${{\mathrm{2Na}}_{\mathrm{}}}^{\mathrm{+}}$ (aq) + ${{\mathrm{2NO}}_3}^{\mathrm{-}}$ (aq)

Cancel the spectator ions or the same ions in the equation

Answer: ${\mathrm{Ba}}^{\mathrm{2+}}$(aq) + ${{\mathrm{SO}}_4}^{\mathrm{2-}}$ (aq) → $\mathrm{Ba}{\mathrm{SO}}_4$ (s) +

(b) $\mathrm{NaOH}$(aq) + $\mathrm{HCl}$ (aq) → $\mathrm{NaCl}$(aq) + $H_{2}O$ (l)

= ${\mathrm{Na}}^{\mathrm{+}}$(aq) + ${\mathrm{OH}}^{\mathrm{-}}$(aq) + $H^{\mathrm{+}}$(aq) + ${\mathrm{Cl}}^{\mathrm{-}}$(aq) → ${\mathrm{Na}}^{\mathrm{+}}$(aq) + ${\mathrm{Cl}}^{\mathrm{-}}$(aq) $H_{2}O$ (l)

From the above equation Cancel the spectator ions or the same ions in the equation

Answer: ${\mathrm{OH}}^{\mathrm{-}}$(aq) + $H^{\mathrm{+}}$(aq) → $H_{2}O$ (l)

Stoichiometric calculations

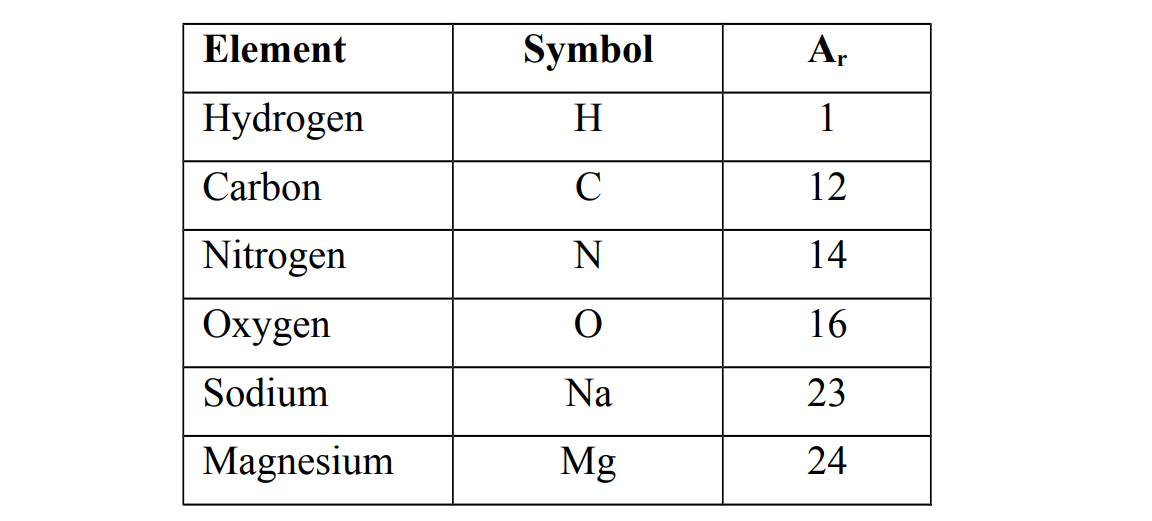
Relative atomic mass

Symbol: $A_{\mathrm{r}}$

Units: It has no units

Relative atomic mass of an element is the mass of one atom of an element compared to 1/12 the mass of carbon−12isotope.

Relative atomic masses of some elements

Relative molecular mass

Symbol: $M_{\mathrm{r}}$

Units: It has no units

Relative molecular mass of a compound is the mass of one molecule of the compound or element compared with 1/12 the mass of carbon−12isotope

Relative molecular mass can also be defined as the sum of relative atomic masses

Example 5

Find the relative molecular, $M_{\mathrm{r}}$ , of the following:

(a) Hydrogen chloride, HCl

(b) Carbon dioxide, ${\mathrm{CO}}_2$

(c) Sodium sulphate, ${\mathrm{Na}}_2{\mathrm{SO}}_4$

(d) Copper (II) sulphate -5-water, CuSO4.5H2O ${\mathrm{CuSO}}_4.{\mathrm{5H}}_{2}O$

Solution

(a) $M_{\mathrm{r}}$ of HCl = (1 x 1) + (1 x 35.5)

= 1 + 35.5

Answer: 36.5

(b) $M_{\mathrm{r}}$ of ${\mathrm{CO}}_2$ = (1 x 12) + (2 x 16)

= 12 + 32

Answer: 44

(c) $M_{\mathrm{r}}$ of ${\mathrm{Na}}_2{\mathrm{SO}}_4$ = (2 x 23) + (1 x 32) + (4 x 16)

46 + 32 + 64

Answer: 142

(d) $M_{\mathrm{r}}$ of ${\mathrm{CuSO}}_4.{\mathrm{5H}}_{2}O$ = (1 x 64) + (1 x 32) + (4 x 16) + (10 x 1) + (5 x 16)

= 64 + 32 + 64 + 10 + 80

Answer: 250

Molar mass

Molar mass is the mass of one mole of a substance

Symbol: MM

SI unit: gram per mole, g/mol

Molar mass can also be defined as relative molecular mass expressed in grams per mole

Example 6

1 mole of potassium (K), MM = (1x39) = 39g/mol

1 mole of sodium (Na), MM = (1x23) = 23g/mol

1 mole of carbon dioxide MM = [(1x12) + (2x16)] = 44g/mol

Mole

The mole is the amount of substance which contains as many elementary entities as they are in 12.00g of carbon-12 isotope

How to calculating number of moles

$\mathrm{Number\; of\; moles}=\frac{\mathrm{mass}}{\mathrm{molar\; mass}}$

$n=\frac{m}{\mathrm{MM}}$

n = mole [mol]

m = mass [g]

MM = molar mass [g/mol]

Example 7

(a) How many moles of potassium are there in 3.9g of potassium?

Solution

$n=\frac{m}{\mathrm{MM}}$

n = ?, m = 3.9g, MM = 39g/mol

$n=\frac{\mathrm{3.9}}{39}$

Answer: $n=\mathrm{0.1\; mol}$

(b) Find the mass of 0.2moles of ammonia molecules, ${\mathrm{NH}}_3$ .

Solution

$n=\frac{m}{\mathrm{MM}}$

n = 0.2 mol, m = ? , MM = 17g/mol

$\mathrm{0.2}=\frac{m}{17}$

$m=\mathrm{0.2}\times 17$

Answer: $m=\mathrm{3.4\; g}$

(c) How many moles of hydrogen atoms does 3.2g of methane, ${\mathrm{CH}}_4$ contains

Solution

MM for ${\mathrm{CH}}_4$ = (1 x 12) + (4 x 1) = 16g/mol

$n=\frac{m}{\mathrm{MM}}$

n = ? , m = 3.2g, MM = 16g/mol

$n=\frac{\mathrm{3.2g}}{\mathrm{16g/mol}}$

$n=\mathrm{0.2\; mol}$

Number of moles of hydrogen in ${\mathrm{CH}}_4$ = number of hydrogen atoms in ${\mathrm{CH}}_4\times \mathrm{n}$

= 4 x 0.2mol

Answer: 0.8mol

Avogadro’s number

Avogadro’s number is the number of particles in exactly one mole of a pure substance 12.00g of carbon contains as many as $\mathrm{6.02}\times {\mathrm{10}}^{23}$ atoms

Alternative term: Avogadro’s constant

Symbol: $N_{\mathrm{A}}$

$N_{\mathrm{A}}=\mathrm{6.02}\times {\mathrm{10}}^{23}$

1 mole of any element has mass equivalent to its mass number and contains $\mathrm{6.02}\times {\mathrm{10}}^{23}$ particles.

Example 8

1 mole of Ca = 40g = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ particles

1 mole of Fe = 56g = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ particles

1 mole of Mg = 24g = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ particles

Elementary entities include atoms, molecules, ions, electrons, protons and neutrons

1 mole of atoms = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ atoms

1 mole of molecules = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ molecules

1 mole of ions = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ ions

1 mole of electrons = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ electrons

1 mole of protons = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ protons

1 mole of neutrons = $\mathrm{6.02}\times {\mathrm{10}}^{23}$ neutrons

Example 9

(a) How many atoms of iron (Fe) are there in 25g of iron?

Solution

56g is the mass number of iron

56g → $\mathrm{6.02}\times {\mathrm{10}}^{23}$ atoms

25g → x

cross multiply to find the answer

Answer: $\mathrm{2.69}\times {\mathrm{10}}^{23}$ Atoms

(b) What is the mass of $\mathrm{1.2}\times {\mathrm{10}}^{23}$ atoms of calcium?

Solution

40g is the mass number of calcium

40g → $\mathrm{6.02}\times {\mathrm{10}}^{23}$ atoms

x → $\mathrm{1.2}\times {\mathrm{10}}^{23}$ atoms

cross multiply to find the answer

Answer: $x=\mathrm{7.97g}$

Mole to mole calculations

Example 10

(a) How many moles of chlorine are required to react 2.5 moles of calcium to produce calcium chloride?

Solution

$\mathrm{Ca}(s)+{\mathrm{Cl}}_2(g)\to {\mathrm{CaCl}}_2(s)$

1 mol Ca → 1 mol ${\mathrm{Cl}}_2$

2.5mol Ca → x

cross multiply to find the answer

Answer: $x=\mathrm{2.5\; mol}$

(b) How many moles of carbon dioxide (${\mathrm{CO}}_2$) will be produced by complete combustion of 2 moles of glucose ( $C_6{H}_{12}{O}_6$) according to the equation?

$C_6{H}_{12}{O}_6+{\mathrm{6O}}_2\to {\mathrm{6CO}}_2+{\mathrm{6H}}_{2}O$

Solution

1 mol $C_6{H}_{12}{O}_6$ → 6 mol ${\mathrm{CO}}_2$

2 mol $C_6{H}_{12}{O}_6$ → x

cross multiply to find the answer

Answer: $x=\mathrm{12\; mol}{\mathrm{CO}}_2$

Mole to mass calculations

Example 11

(a) What mass of hydrogen can be produced by reacting 6 moles of aluminium with hydrochloric acid?

$\mathrm{2Al(s)}+\mathrm{6HCl(aq)}\to {\mathrm{2AlCl}}_3+{\mathrm{3H}}_2(g)$

Solution

2 mol → 6g $H_2$

6 mol Al → x

cross multiply to find the answer

Answer: $x=\mathrm{18\; g}{H}_2$

(b) How many grams of oxygen are required to react with 0.3 moles of aluminium to produce aluminium oxide?

$\mathrm{4Al\; (s)}+{\mathrm{3O}}_2(g)\to {\mathrm{2Al}}_2{O}_3(s)$

Solution

4 mol Al → 96g $O_2$

0.3 mol Al → x

cross multiply to find the answer

Answer: $x=\mathrm{7.2\; g}{O}_2$

Mass to mass calculation

Example 12

(a) Calculate the mass of calcium chloride produced when 40g of calcium carbonate reacts with hydrochloric acid

${\mathrm{CaCO}}_3(s)+\mathrm{2HCl}(aq)\to {\mathrm{CaCl}}_2(aq)+H_{2}O(l)+{\mathrm{CO}}_3(g)$

Solution

100g ${\mathrm{CaCO}}_3$ → 111g ${\mathrm{CaCl}}_2$

40g ${\mathrm{CaCO}}_3$ → x

cross multiply to find the answer

Answer: $x=\mathrm{44.4g}{\mathrm{CaCl}}_2$

(b) How many tonnes of uranium can be produced in the above reaction using 24 tonnes of magnesium?

${\mathrm{UF}}_4+\mathrm{2Mg}\to {\mathrm{2MgF}}_2+U$

Solution

48g Mg → 238g U

24tonnes Mg → x

cross multiply to find the answer

$x=\frac{\mathrm{24\; tonnes\; Mg\; x\; 238g\; U}}{\mathrm{48g\; Mg}}$

Answer: $x=\mathrm{119\; tonnes\; U}$

Limiting reagent

A limiting reagent is a reactant that is in short supply by the mole ratio and hence it finishes before the other reactants are completely reacted.

A limiting reagent is always smaller or in less quantity compared to the other reactant

A limiting reagent is found by dividing the number of moles of each reactant by its stoichiometric coefficient in balanced chemical equation.

A limiting reagent determines the extent to which the chemical reaction can proceed and the amount of products that would be formed. Once the limiting reagent is finished, the reaction stops even if the other reactants are still available in the reaction vessel.

For this reason, it is important to identify the limiting reagent before calculating the theoretical yield

In the identification of the limiting reagent

1. The balanced chemical equation and the mole ratio of reactants are used
2. You cannot use volumes, concentrations or masses of the reactants since these will easily mislead you.

Note An excess reagent is a reactant that remains unreacted at the end of the reaction.

Example 13

19.5g of zinc and 9.40g of sulphur were heated together

Zn(s) + S(s) → ZnS(s)

(a) Which of the two is the limiting reactant?

(b) How many moles of zinc remain unreacted?

(c) How many grams of zinc element remain unreacted?

(d) Calculate the mass of zinc sulphide formed

Solution

(a)

$\mathrm{n(Zn)}=\frac{m}{\mathrm{MM}}$    $\mathrm{n(S)}=\frac{m}{\mathrm{MM}}$

$=\frac{\mathrm{19.5g}}{\mathrm{65g/mol}}$    $=\frac{\mathrm{9.40g}}{\mathrm{32g/mol}}$

$=\mathrm{0.3\; mol}$    $=\mathrm{0.29\; mol}$

The limiting reagent is sulphur because it has a smaller number of moles compared to zinc.

(b)

Number of moles of Zn unreacted = 0.3mol – 0.29mol

$=\mathrm{0.01\; mol}$

(c)

m = n x MM

m = 0.01mol x 65g/mol

m = 0.65g of Zn remained unreacted

(d)

32g S → 97g ZnS

9.4g S → x

$x=\frac{\mathrm{9.40\; g\; S\; x97\; g\; ZnS}}{\mathrm{32g\; S}}$

Answer: x = 28.5g ZnS

Gas volume (Molecular volume of gases)

Avogadro’s law

The law states that: the volume of a gas is directly proportional to the number of moles of the gas molecules present if the pressure and temperature are constant

$\mathrm{Number\; of\; moles}=\frac{\mathrm{Volume}}{\mathrm{Molar\; volume}}$

$n=\frac{V}{\mathrm{Vm}}$

n = number of moles [mol]

V = volume [cm3] or [dm3]

Vm = molar volume [cm3/mol] or [dm3/mol]

Room temperature and pressure (r.t.p): The volume of one mole of any gas is 24dm3 or 24000cm3 at r.t.p

Standard temperature and pressure (s.t.p): The volume of one mole of any gas is 22.4dm3 or 22400cm3 at s.t.p

Example 14

(a) Calculate the number of moles of carbon dioxide molecules present in 240cm3 of gas at r.t.p

Solution

$n=\frac{V}{\mathrm{Vm}}$

$n=\frac{\mathrm{240\; cm3}}{\mathrm{24000\; cm3/mol}}$

Answer: n = 0.01 mol

(b) What volume of hydrogen measured at s.t.p is produced when 0.35g of Lithium reacts with water?

$\mathrm{2Li(s)}+{\mathrm{2H}}_2\mathrm{O\; (l)}\to \mathrm{2LiOH(aq)}+H_2(g)$

Solution

$\mathrm{n\; (Li)}=\frac{V}{\mathrm{Vm}}$

$n=\frac{\mathrm{0.35g}}{\mathrm{7g/mol}}$

n = 0.05mol

2 mol Li → 1mol $H_2$

0.05mol Li → x

$n=\frac{\mathrm{0.05\; molLi\; \times \; 1\; mol\; H2}}{\mathrm{2\; mol\; Li}}$

n = 0.025mol $H_2$

V = n x Vm

V = 0.025mol x 22.4dm3/mol

Answer: V = 0.56dm3

Relative molecular mass of gases

$\mathrm{Density}=\frac{\mathrm{mass}}{\mathrm{volume}}$

Example 15

The density of a gas is 0.71g/dm3 at r.t.p. What is the mass of the gas?

Solution

Mass = Density x volume

= 0.71g/dm3 x 24dm3

= 17.04g

Concentration

Concentration is the amount of solute dissolved in a unit volume of the solution.

The concentration expressed in mol/dm3 is called molarity.

$M=\frac{n}{\mathrm{v}}$

M = Molarity [mol/dm3]Molarity is the number of moles of solute in one liter of solution

n = moles [mol]

V = volume [dm3]

Example 16

(a) A solution of glucose contains 0.45g of glucose in 0.075dm3 of solution. Calculate the concentration of glucose solution in g/dm3 .

Solution

$M=\frac{n}{\mathrm{v}}$

$=\frac{\mathrm{0.45g}}{0.075}$

Answer: 6.0g/dm3